So far we've been looking at graphs of relationships between two variables. In these relationships, the value of one of the variables would be determined by the value of another, for instance, total production costs being determined by how much is produced. Sometimes we want to show all the possible combinations of two variables that give some common value. In the diagram to the right the value of A does not determine the value of B, instead, we've shown the set of all the (positive) values of A and B that add up to 30.

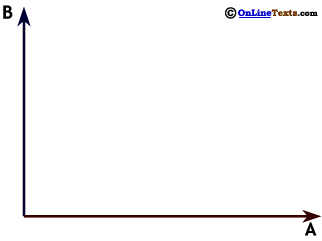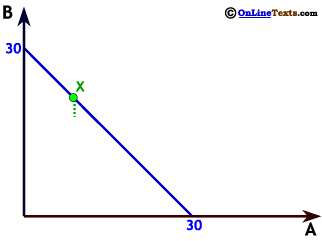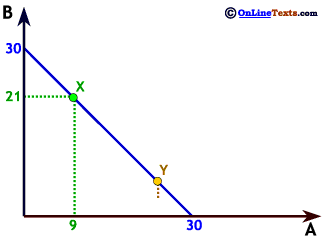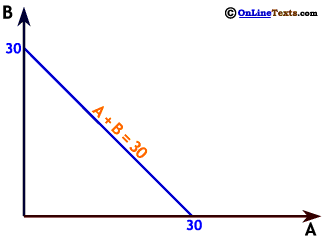
    Points X and Y are two of the points where A + B = 30.¹⁰ At point X the value of A is 9 and the value of B is 21 (9 + 21 = 30). At point Y the value of A is 24 and the value of B is 6 (24 + 6 = 30). The endpoints of the line work too. On the vertical axis the line crosses where B is 30 and A is zero (30 + 0 = 30), and on the horizontal axis A is 30 and B is zero (0 + 30 = 30).


¹⁰ If we allow fractional values and/or negative numbers, there are an infinite number of points where A + B = 30.

Click Here if you don't see topics listed to the left and below.